Plate 3-4

CALCULATION OF GFR

In clinical practice, it is not possible to measure hydrostatic or oncotic pressure in the glomerular capillaries or in Bowman’s space. Instead, the GFR can be determined based on the rate at which specific molecular markers enter the urine. For all solutes,

filtration rate + secretion rate − reabsorption rate = excretion rate

For a solute x that is freely filtered at the glomerulus, then neither reabsorbed nor secreted:

filtration rate = excretion rate

[P]_x × GFR = 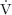× [U]_x

[P]_x = concentration of x in plasma

GFR = glomerular filtration rate

[U]_x = concentration of x in urine

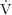= rate of urine production

Rearranging, and recalling the clearance formula introduced previously, it is evident that:

C(x) = GFR

Thus for a solute x that is freely filtered and then neither reabsorbed nor secreted, the volume of plasma cleared of x per unit of time is equal to the volume of plasma filtered per unit of time.

Inulin, a fructose polysaccharide, is an example of a substance that is filtered but neither reabsorbed nor secreted. Therefore, calculating its clearance provides a reasonable approximation of the GFR.

In the clinical setting, however, it is often impractical to measure the clearance of inulin, since it is an exogenous substance that must be administered until steadystate concentrations are reached. Moreover, there is a risk of anaphylactoid reactions. Instead, creatinine clearance (Ccr) is calculated, since creatinine is an endogenous chemical that is filtered at the glomerulus, undergoes little secretion, and is usually at a steady-state concentration in plasma. It originates from creatine and phosphocreatine in skeletal muscle, which are normally metabolized at a relatively constant rate. Creatinine clearance provides a close approximation of the GFR, but it tends to overestimate by up to 10% to 20%, owing to the small amount of creatinine secretion. This error increases when the GFR is low, since secretion then accounts for a relatively greater fraction of creatinine’s overall clearance.

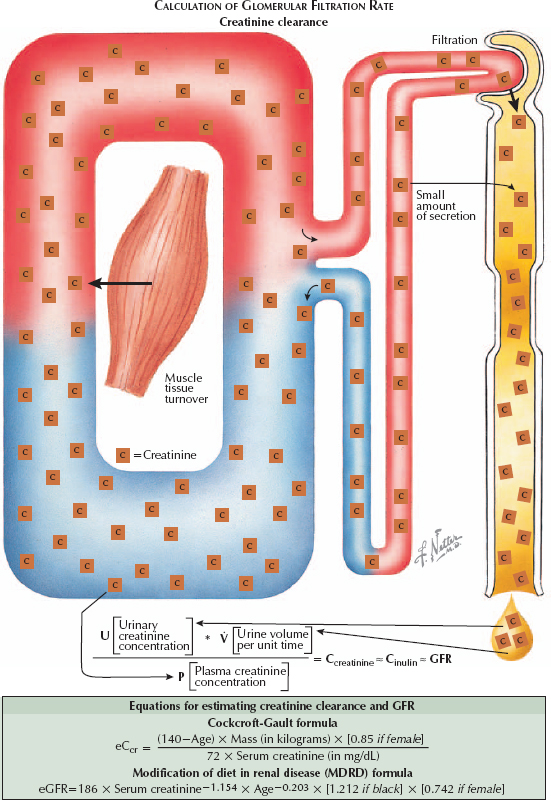
To obtain a precise value for creatinine clearance, and thus a close estimate of the GFR, one could collect a 24-hour urine sample and solve for Ccr as *[U]_Cr/ [P]_Cr. Because of the inconvenience of 24-hour collections, however, most clinicians instead rely on regression equations that estimate Ccr based on [P]_Cr. These estimates use age, sex, weight, and race as corrective factors, since an individual’s normal creatinine concentration depends on his or her muscle mass. Examples include the Cockcroft-Gault and MDRD formulas.

The average GFR in a normal individual is 120 to 130 mL/minute. Because the relationship between GFR and [P]_Cr is inverse and exponential, a doubling of [P]_Cr indicates the GFR has declined by half. A decline in the GFR to abnormally low values is known as renal insufficiency; if it occurs over a short time period, it is known as acute kidney injury (or acute renal failure, see Plate 4-1); if it is sustained, it is known as chronic kidney disease (or chronic renal failure, see Plate 4-66).

By calculating GFR/RPF, one can obtain the filtration fraction, defined as the fraction of plasma reaching the glomerular capillaries that is filtered into Bowman’s space. Normal values for filtration fraction are around 20%.